How To Find Mean Median And Mode

Mean Mode Median

Hateful, median, and mode are the 3 measures of key tendency in statistics. We identify the cardinal position of whatsoever data fix while describing a set up of information. This is known equally the mensurate of primal trend. We encounter data every day. Nosotros discover them in newspapers, manufactures, in our bank statements, mobile and electricity bills. The listing is endless; they are present all around us. At present the question arises if nosotros tin figure out some of import features of the data past considering simply certain representatives of the information. This is possible by using measures of central tendency or averages, namely hateful, median, and mode.

Let us understand mean, median, and manner in detail in the following sections using solved examples.

1.	Mean, Median, and Manner in Statistics
2.	Mean
3.	Median
4.	Mode
5.	Mean, Median, and Mode Formulas
6.	Relation between Hateful, Median, and Mode
7.	Difference between Mean and Boilerplate
8.	Difference between Mean and Median
9.	FAQs on Hateful, Median, and Way

Mean, Median and Mode in Statistics

Mean, median, and mode are the measures of central tendency, used to study the various characteristics of a given set of data. A measure of central trend describes a set of data past identifying the central position in the information ready as a unmarried value. We can think of it as a tendency of data to cluster effectually a middle value. In statistics, the iii most common measures of fundamental tendencies are Mean, Median, and Mode. Choosing the best mensurate of central tendency depends on the type of data we take.

Permit's begin by understanding the significant of each of these terms.

Mean

The arithmetic mean of a given data is the sum of all observations divided by the number of observations. For example, a cricketer's scores in five ODI matches are as follows: 12, 34, 45, fifty, 24. To detect his average score in a match, we calculate the arithmetic mean of data using the hateful formula:

Mean = Sum of all observations/Number of observations

Mean = (12 + 34 + 45 + 50 + 24)/5

Mean = 165/5 = 33

Mean is denoted by x̄ (pronounced as x bar).

Types of Data

Data tin exist nowadays in raw form or tabular grade. Let's discover the hateful in both cases.

Raw Data

Let \(x_{1}\), \(x_{ii}\), \(x_{3}\) ……\(x_{due north}\) be north observations.

We can find the arithmetic mean using the hateful formula.

Mean, x̄ = \(\dfrac{x_1+x_2+...x_n}{n}\)

Instance: If the heights of v people are 142 cm, 150 cm, 149 cm, 156 cm, and 153 cm.

Detect the mean tiptop.

Mean pinnacle, x̄ = (142 + 150 + 149 + 156 + 153)/five
= 750/5
= 150

Mean, x̄ =150 cm

Thus, the mean height is 150 cm.

Frequency Distribution (Tabular) Form

When the information is present in tabular form, we use the following formula:

Hateful, x̄ = \(\dfrac {x_1f_1 + x_2f_2+....x_nf_n}{\ f_+ f_2+.....f_n}\)

Consider the following example.

Example 1: Find the mean of the following distribution:

x	4	6	9	x	15
f	5	10	x	7	eight

Solution:

Calculation tabular array for arithmetic hateful:

ten\(_i\)	f\(_i\)	x\(_i\)f\(_i\)
iv	five	20
6	10	threescore
9	x	ninety
x	seven	70
15	8	120
	\( \sum f_i=40\)	\( \sum x_i f_i=360\)

Mean, x̄ = \(\dfrac{\sum x_if_i}{\sum f_i}\)
= 360/40
= 9

Thus, Mean = ix

Example two: Hither is an example where the information is in the grade of course intervals. The post-obit table indicates the information on the number of patients visiting a infirmary in a month. Find the average number of patients visiting the hospital in a day.

Number of patients	Number of days visiting infirmary
0-x	2
10-twenty	6
twenty-30	9
xxx-40	7
40-50	4
l-threescore	2

Solution:

In this example, we notice the classmark (as well called as mid-point of a class) for each grade.

Note: Class marker = (lower limit + upper limit)/2

Let \(x_{i}\), \(x_{2}\), \(x_{3}\) ……\(x_{northward}\) be the course marks of the corresponding classes.

Hence, we become the post-obit tabular array:

Course mark (xi)	frequency (fi)	10ifi
5	ii	10
xv	6	90
25	9	225
35	7	245
45	4	180
55	2	110
Total	\(\sum f_i=30\)	\(\sum f_ix_i=860\)

Hateful, x̄ = \(\dfrac{\sum x_if_i}{\sum f_i} \)
= 860/30
= 28.67

x̄ = 28.67

Challenging Question:

Permit the mean of \(x_{one}\), \(x_{2}\), \(x_{3}\) ……\(x_{n}\) be A, so what is the mean of:

• (\(x_{one}\) + thousand) ,(\(x_{two}\) + yard), (\(x_{3}\) + one thousand), ……(\(x_{n}\) + thousand)
• (\(x_{one}\) - k) ,(\(x_{2}\) - k), (\(x_{3}\) - grand), ……(\(x_{n}\) - k)
• k\(x_{1}\), g\(x_{ii}\), one thousand\(x_{3}\) ……yard\(x_{n}\)

Median

The value of the middlemost ascertainment, obtained after arranging the information in ascending society, is called the median of the data.

For example, consider the data: 4, 4, 6, three, ii. Permit's suit this information in ascending order: 2, iii, 4, 4, half dozen. There are v observations. Thus, median = middle value i.e. 4. Nosotros can see here: 2, 3, 4, 4 , 6 (Thus, 4 is the median)

Instance 1: Ungrouped Information

• Stride ane: Adapt the data in ascending or descending social club.
• Pace 2: Let the total number of observations exist northward.

To find the median, nosotros need to consider if due north is even or odd. If northward is odd, then use the formula:

Median = (north + 1)/2^th observation

Example 1: Allow'due south consider the data: 56, 67, 54, 34, 78, 43, 23. What is the median?

Solution:

Arranging in ascending lodge, nosotros get: 23, 34, 43, 54, 56, 67, 78. Hither, north (no.of observations) = 7

So, (7 + i)/ii = 4

∴ Median = 4^thursday ascertainment

Median = 54

If due north is fifty-fifty, and then use the formula:

Median = [(n/2)^thursday obs.+ ((n/2) + 1)^th obs.]/2

Example ii: Let'south consider the information: fifty, 67, 24, 34, 78, 43. What is the median?

Solution:

Arranging in ascending order, we get: 24, 34, 43, 50, 67, 78.

Here, n (no.of observations) = half dozen

half dozen/2 = three

Using the median formula,

Median = (3^rd obs. + 4^th obs.)/2

= (43 + 50)/ii

Median = 46.five

Case 2: Grouped Information

When the data is continuous and in the form of a frequency distribution, the median is found equally shown below:

Footstep 1: Notice the median class.

Let n = total number of observations i.e. \(\sum f_i \)

Note: Median Course is the class where (northward/two) lies.

Step ii: Employ the post-obit formula to find the median.

Median = \( l + [\dfrac {\dfrac{n}{2}-c}{f}]\times h\)

where,

• l = lower limit of median class
• c = cumulative frequency of the grade preceding the median grade
• f = frequency of the median class
• h = class size

Let'due south consider the following example to understand this better.

Instance: Find the median marks for the following distribution:

Classes	0-10	10-20	20-30	30-40	40-50
Frequency	2	12	22	8	half dozen

Solution:

We need to calculate the cumulative frequencies to discover the median.

Adding tabular array:

Classes	Number of students	Cumulative frequency
0-ten	ii	ii
10-xx	12	2 + 12 = 14
20-xxx	22	14 + 22 = 36
30-40	8	36 + 8 = 44
40-50	half dozen	44 + six = fifty

N = 50

N/2 = fifty/2 = 25

Median Class = (20 - thirty)

l = 20, f = 22, c = 14, h = 10

Using Median formula:

Median = \(l + [\dfrac {\dfrac{n}{ii}-c}{f}]\times h\)

= 20 + (25 - fourteen)/22 × 10

= 20 + (11/22) × 10

= 20 + 5 = 25

∴ Median = 25

Mode

The value which appears virtually often in the given information i.e. the observation with the highest frequency is called a mode of information.

Case one: Ungrouped Data

For ungrouped data, we just need to identify the ascertainment which occurs maximum times.

Mode = Observation with maximum frequency

For instance in the data: half dozen, viii, 9, 3, iv, 6, 7, 6, 3 the value 6 appears the most number of times. Thus, mode = 6. An easy way to think way is: Most Often Data Entered. Note: A information may take no mode, 1 manner, or more than one manner. Depending upon the number of modes the data has, information technology tin can be chosen unimodal, bimodal, trimodal, or multimodal.

The example discussed above has only 1 mode, then it is unimodal.

Case two: Grouped Data

When the information is continuous, the mode can exist found using the following steps:

• Step i: Find modal class i.eastward. the class with maximum frequency.
• Step 2: Discover mode using the following formula:

Mode = \(l + [\dfrac {f_m-f_1}{2f_m-f_1-f_2}]\times h\)

where,

• l = lower limit of modal grade,
• \( f_m =\) frequency of modal class,
• \( f_1=\) frequency of form preceding modal course,
• \( f_2= \) frequency of grade succeeding modal class,
• h = course width

Consider the post-obit example to empathise the formula.

Instance: Observe the mode of the given information:

Marks Obtained	0-20	twenty-40	40-60	60-80	eighty-100
Number of students	five	10	12	vi	3

Solution:

The highest frequency = 12, so the modal grade is 40-60.

l = lower limit of modal form = xl

\( f_m\) = frequency of modal class = 12

\( f_1\) = frequency of class preceding modal class = 10

\( f_2\) = frequency of class succeeding modal class = 6

h = class width = 20

Using the manner formula,

Way = \(l + [\dfrac {f_m-f_1}{2f_m-f_1-f_2}]\times h\)

= 40 + \([\dfrac{12-x}{ii \times 12 - ten-6} ]\times 20\)

= twoscore + (ii/8) × 20

= 45

∴ Mode = 45

Mean, Median and Mode Formulas

We covered the formulas and method to find the mean, median, and mode for grouped and ungrouped set of information. Allow u.s. summarize and call back them using the listing of mean, median, and mode formulas given beneath,

Mean formula for ungrouped data: Sum of all observations/Number of observations

Mean formula for grouped data: x̄ = \(\dfrac {x_1f_1 + x_2f_2+....x_nf_n}{\ f_+ f_2+.....f_n}\)

Median formula for ungrouped data: If northward is odd, so use the formula: Median = (n + one)/two^thursday observation. If n is fifty-fifty, then apply the formula: Median = [(northward/two)^thursday obs.+ ((n/2) + 1)^th obs.]/2

Median formula for grouped data: Median = \( l + [\dfrac {\dfrac{n}{two}-c}{f}]\times h\)

where,

• fifty = lower limit of median class
• c = cumulative frequency of the grade preceding the median class
• f = frequency of the median class
• h = grade size

Mode formula for ungrouped information: Mode = Observation with maximum frequency

Mode formula for grouped data: Mode = \(fifty + [\dfrac {f_m-f_1}{2f_m-f_1-f_2}]\times h\)

where,

• fifty = lower limit of modal form,
• \( f_m\) = frequency of modal course,
• \( f_1\) = frequency of form preceding modal class,
• \( f_2\) = frequency of form succeeding modal class,
• h = class width

Relation Between Mean, Median and Manner

The three measures of fundamental values i.due east. mean, median, and manner are closely connected by the following relations (called an empirical relationship).

2Mean + Mode = 3Median

For instance, if we are asked to calculate the mean, median, and mode of continuous grouped information, then we can summate mean and median using the formulas as discussed in the previous sections and and then find mode using the empirical relation.

For example, we have data whose way = 65 and median = 61.6.

And so, nosotros tin can observe the mean using the above mean, median, and fashion relation.

2Mean + Style = three Median

∴2Mean = 3 × 61.half dozen - 65

∴2Mean = 119.8

⇒ Mean = 119.8/ii

⇒ Mean = 59.ix

Difference Between Mean and Boilerplate

The term average is frequently used in everyday life to denote a value that is typical for a group of quantities. Boilerplate rainfall in a calendar month or the average age of employees of an organization is typical examples. Nosotros might read an commodity stating "People spend an average of 2 hours every day on social media. " We understand from the use of the term average that non everyone is spending 2 hours a day on social media just some spend more than time and some less.

However, we tin can understand from the term average that two hours is a adept indicator of the amount of fourth dimension spent on social media per solar day. Well-nigh people utilize average and mean interchangeably even though they are not the aforementioned.

• Boilerplate is the value that indicates what is most probable to exist expected.
• They aid to summarise large data into a single value.

An average tends to lie centrally with the values of the observations arranged in ascending order of magnitude. So, nosotros telephone call an average measure of the central tendency of the information. Averages are of unlike types. What we refer to as hateful i.due east. the arithmetics mean is one of the averages. Hateful is called the mathematical average whereas median and mode are positional averages.

Deviation Between Hateful and Median

Mean is known as the mathematical average whereas the median is known as the positional average. To understand the deviation between the 2, consider the following example. A section of an organization has v employees which include a supervisor and four executives. The executives draw a salary of ₹10,000 per month while the supervisor gets ₹forty,000.

Mean = (10000 + 10000 + 10000 + 10000 + 40000)/five = 80000/5 = 16000

Thus, the mean salary is ₹sixteen,000.

To observe the median, we consider the ascending society: 10000, 10000, 10000, 10000, 40000.

n = 5,

so, (n + ane)/2 = iii

Thus, the median is the 3rd ascertainment.

Median = 10000

Thus, the median is ₹10,000 per month.

Now let us compare the two measures of central tendencies.

We tin observe that the hateful salary of ₹16,000 does not give even an estimated salary of whatsoever of the employees whereas the median salary represents the data more finer.

One of the weaknesses of hateful is that it gets afflicted by farthermost values.

Look at the post-obit graph to understand how farthermost values touch mean and median:

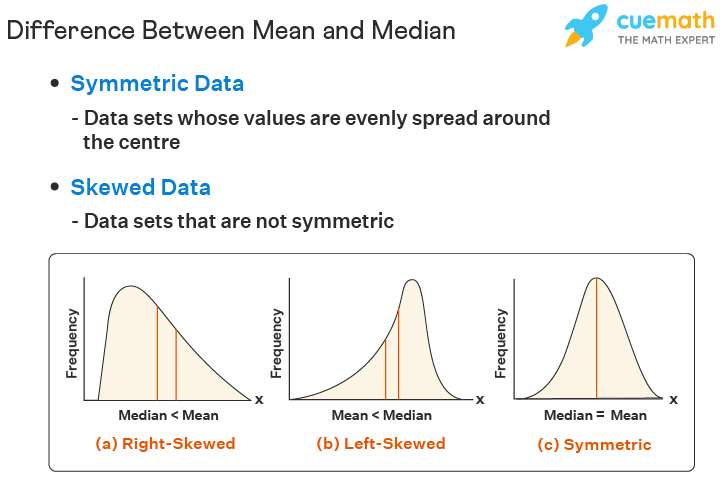
So, mean is to be used when we don't have extremes in the data.

If we have extreme points, then the median gives a improve estimation.

Here's a quick summary of the differences between the 2.

Hateful Vs Median	Mean	Median
Definition	Average of given data (Mathematical Average)	Key value of data (Positional Average)
Calculation	Add all values and divide by total number of observations	Adjust data in ascending / descending order and find middle value
Values of data	Every value is considered for calculation	Every value is not considered
Effect of extreme points	Greatly effected by extreme points	Doesn't get effected by farthermost points

Related Topics on Hateful, Median, and Way:

• Average
• Categorical Data
• Range in Statistics
• Geometric Mean

Solved Examples on Mean, Median and Way

1. Example 1: If the mean of the post-obit data is 20.6, find the missing frequency (p).

   10	10	15	20	25	35
   f	3	ten	p	seven	5

   Solution:

   Let u.s.a. make the calculation table for this :

   10\(_i\)	f\(_i\)	10\(_i\)f\(_i\)
   ten	iii	x × 3 = 30
   15	10	15 × 10 = 150
   20	p	20 × p = 20p
   25	7	25 × 7 = 175
   35	v	35 × 5 = 175
   Total:	\(\sum {f_i}\) = 25 + p	\(\sum {f_ix_i}\) = 530 + 20p

   Mean = \(\dfrac{\sum f_ix_i}{\sum f_i} \)
   twenty.six = (530 + 20p)/(25 + p)
   530 + 20p = 515 + 20.6p
   15 = 0.6p
   p =15/0.6
   = 25

   ∴ The missing frequency (p) = 25

2. Example 2: The mean of 5 numbers is 18. If ane number is excluded, their mean is sixteen. Find the excluded number.

   Solution:

   Given, n = 5, x̄ = 18

   x̄ = \(\dfrac{\sum {x_i}}{n}\)

   \(\sum {x_i}\)= v × eighteen = 90

   Thus, the total of v numbers = 90

   Let the excluded number exist "a".

   Therefore, total of 4 numbers = 90 - a

   Hateful of 4 numbers = (90 - a)/4
   xvi = (ninety - a)/iv
   90 - a = 64
   a = 26

   ⇒ The missing number is 26.

3. Example 3: A survey on the heights (in cm) of fifty girls of form X was conducted at a school and the post-obit data were obtained:

   Height (in cm)	120-130	130-140	140-150	150-160	160-170	Total
   Number of girls	2	8	12	xx	8	50

   Detect the mode and median of the above data.

   Solution:

   Modal class = 150 - 160

   [as it has maximum frequency]

   l = 150, h = x, \(f_m\) = 20, \(f_1\) = 12, \(f_2\) = 8

   Mode = \(50 + [\dfrac {f_m-f_1}{2f_m-f_1-f_2}]\times h\) = 150 + (20 - 12)/(two × twenty - 12 - viii) × 10 = 150 + 4 = 154

   ∴ Way = 154

   To find the median, we need cumulative frequencies.

   Consider the table:

   Class Intervals	No. of girls (fi)	Cumulative frequency (c)
   120-130	two	2
   130-140	8	2 + 8 = 10
   140-150	12 = f\(_1\)	x + 12 = 22 (c)
   150-160	twenty = f\(_m\)	22 + 20 = 42
   160-170	8 = f\(_2\)	42 + 8 = fifty (northward)

   n = 50
   ⇒due north/ii = 25

   ∴ Median class = (150 - 160)

   l = 150, c = 22, f = xx, h = 10

   Median = l + [(due north/2 - c)/f] × h = 150 + [((50/2) - 22)/twenty] × x = 150 + i.5 = 151.5

   ∴ Way = 154, Median = 151.v

get to slidego to slidego to slide

Breakdown tough concepts through simple visuals.

Math volition no longer be a tough bailiwick, peculiarly when you understand the concepts through visualizations.

Book a Gratis Trial Form

Practice Questions on Mean, Median, Mode

go to slidego to slide

FAQs on Mean, Median, Fashion

What is Mean, Median, Style in Statistics?

Mean, median, way are measures of central tendency or, in other words, different kinds of averages in statistics. Mean is the "boilerplate", where we observe the total of all the numbers and and then carve up past the number of numbers, while the median is the "heart" value in the listing of numbers. Way is the value that occurs most often in the given set of data.

What are Formulas to Find Mean, Median, and Mode?

Dissimilar sets of formulas can exist used to find hateful, median, and fashion depending upon the type of data if that is grouped or ungrouped. The following formulas can be used to find the hateful median and mode for ungrouped data:

• Mean = Sum of all observations/Number of observations
• If northward is odd, then use the formula: Median = (due north + 1)/two^th observation. If northward is fifty-fifty, so use the formula: Median = [(northward/2)^th obs.+ ((north/2) + i)^th obs.]/2
• Manner = Observation with maximum frequency

How to Find Mean, Median and Mode for Grouped Data?

We tin can discover the mean, mode, and median for grouped data using the below-given formulas,

Mean, x̄ = \(\dfrac {x_1f_1 + x_2f_2+....x_nf_n}{\ f_+ f_2+.....f_n}\)

Median = \( l + [\dfrac {\dfrac{north}{2}-c}{f}]\times h\)
where,

• l = lower limit of median form
• c = cumulative frequency of the course preceding the median course
• f = frequency of the median class
• h = class size

Manner = \(l + [\dfrac {f_m-f_1}{2f_m-f_1-f_2}]\times h\)

where,

• l = lower limit of modal form,
• \( f_m =\) frequency of modal class,
• \( f_1=\) frequency of class preceding modal class,
• \( f_2= \) frequency of class succeeding modal grade,
• h = course width

How to Notice Hateful Median and Mode?

The mean, median, and way for a given prepare of data tin be obtained using the mean, median, formula. Click here to check these formulas in detail and sympathize their applications.

What Does Mean, Way, and Median Represent?

Mean, mode, and median are the three measures of central tendency in statistics. Mean represents the average value of the given ready of information, while the median is the value of the middlemost observation obtained later on arranging the data in ascending gild. Way represents the well-nigh common value. It tells you which value has occurred nigh often in the given information. On a bar chart, the mode is the highest bar. It is used with categorial information such as near sold T-shirts size.

How to Find Median Using Mean Median Style Formula?

Median is the value of the middlemost observation, obtained after arranging the data in ascending gild.

To discover the same, we demand to consider two cases.

If n is odd, and so employ the formula: Median = (n + i)/2th observation.

If northward is even, so use the formula: Median = [(n/ii)th obs.+ ((n/2) + 1)th obs.]/ii

For grouped information, the median is obtained using the median formula:

\(\text {Median= } fifty + [\dfrac {\dfrac{n}{two}-c}{f}]\times h\)

Are Mean, Mode, and Median the Aforementioned?

No, mean, fashion and median are non the same.

• Mean is the boilerplate of the given sets of numbers. We need to add the numbers upwardly so carve up their sum by the number of observations.
• For finding the mode, we observe whether any number appears more than one time. The number which appears almost is the mode. If there are other numbers that repeat to the same level, there may exist more than one mode. A set could be bimodal or trimodal. But the mean of a given data is unique.
• Median is the value of the middlemost ascertainment, obtained afterward arranging the data in ascending society.

Source: https://www.cuemath.com/data/mean-median-mode/

Posted by: bukowskiolow1967.blogspot.com

Share this post